19: Here's A Discount Rate Visual To Consider
Even a modest discount rate will soon put you underwater from a present value perspective
Part of every investment decision - whether explicit or not - is the use of a discount rate to bring future cash flows to the present. While everyone accepts the general concept, not as many have stopped to fully appreciate how hard it is to overcome economic gravity.
One way to visualize the concept is like the ocean floor. The farther away from shore (generally) the farther the ocean floor is from sea level. To bring treasure up from the bottom requires increasingly more effort. So too with bringing future dollars back up to the present.
I’ve been thinking about this a lot lately because I’ve found a company trading for half of where the market valued it just four months ago. Part of why the share price fell is also part of its allure: it’s a growth company. A small stumble led Wall Street to discard its shares and presented a possible buying opportunity. (Paid Watchlist Investing subscribers will learn the name of this company first when the next issue is released.)
As Warren Buffett and Charlie Munger have said, growth is part of the value equation. But that doesn’t make it easy. Growth comes at a cost. It means an investment of present value dollars to secure more in present value terms from future investment. It comes with uncertainty and risks that the future won’t unfold as needed to justify the current share price.
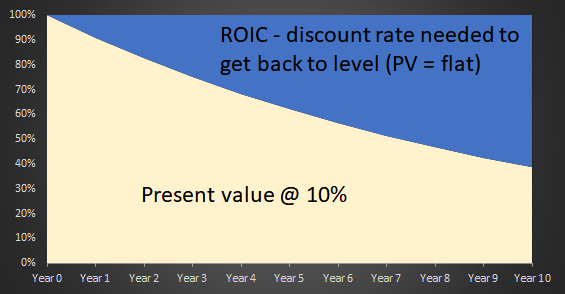
As the chart below illustrates, the discount rate compounds to create a big hurdle to overcome just to get to the surface. A company’s return on capital must be high enough to allow growth to overcome the compounding effects of the discount rate. And that’s just to get to breakeven. If you’re modeling growth in future cash flows in present value terms, that requires even more favorable prospects. It’s not impossible, but it’s not as easy as some analysts and investors seem to indicate.
Does this seem like a good way to visualize discount rates and the challenges of growth? How do you think about these concepts?
Stay rational! -Adam
Check out the paid version of Watchlist Investing, which features Deep Dives and Quick Looks into companies to help you be in a position to move quickly when Mr. Market overreacts and presents buying opportunities.
